The major assumption of Sharpe's single-index model is that all the covariation of security returns can be explained by a single factor. This factor is called the index, hence the name "single-index model." One version of the model, called the market model, uses a market index such as the S&P500 as the factor, although in principle, any factor that influences security returns can serve as the index. Here, we will adopt the terminology associated with the market model.

This independence leads to a significant simplification of the covariance matrix of returns. In particular, the off-diagonal terms in the matrix take on a simple form. Recall, that the covariance between the returns of i and j is

If you substitute the equation

multiply r_i and r_j, and take expectations, you will find that

Similarly, the variance of r_i reduces to:

As you can see, the variance is the sum of two components. The first is the variance arising from the index, which is called the systematic risk. The second is the firm-specific or unsystematic risk.

Since it is assumed that the unsystematic components are independent across firms, the expression for the portfolio variance reduces to

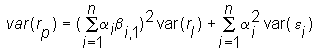
The portfolio problem then simplifies to

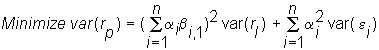
subject to the weights summing to one, and resulting in a desired expected return.

It is instructive to compare the above expression for portfolio variance with the objective function used in the full Markowitz problem:

You can see that there is a considerable saving in the number of terms contained in the single-index model compared to the full Markowitz formulation. For example, with 100 stocks, the Markowitz problem has 10,000 (100x100) variances and covariances (of which only 5050 are distinct because the covariance matrix is symmetric). This compares to 100 betas, 100 residual variances, and 1 variance of the index in the single-index model.

Most of this simplification comes from the assumption that the unsystematic components are independent, in which case the covariance of returns is:

This means that the covariance matrix in the single-index model has the security variance terms down the diagonal and the product of the beta and the variance of the index in the off-diagonal part.

There is a close relationship between the single-index model and the CAPM. To see this, recall that the CAPM implies

where r_M is the return on the market portfolio (see the two-fund theorem in Chapter 7). The market portfolio is more appropriately called the tangency portfolio, since it is the point of tangency between the minimum variance frontier and the capital market line.

If we take the expectation of r_i in the single-index model and substitute

we get

This is the same functional form as in the CAPM, and if the index equals the market portfolio, the result is exactly the same.

On a cautionary note, you should remember first, that the market portfolio in the CAPM is not the same as a "market index." In fact, if you use a market index such as the S&P 500 in the single-index model, it is quite unlikely that it will coincide with the tangency portfolio identified by the CAPM. This will become readily apparent when you use the single-index model to analyze real-world data. Second, you should remember that even though the functional form for the expected return is similar, the single-index model leads to a simplification of the portfolio choice model because of the additional assumption that the idiosyncratic components of return are independent across stocks.

The topic Calculation of the Optimal Portfolio describes how the assumptions of the single-index model allow you to quickly calculate optimal portfolio weights.

(C) Copyright 1999, OS Financial Trading System